
The world of Mathematics is in for a shock because Babylonians were well aware of Pythagorean principles even before Pythagoras did. Analyzing Si.427, a 3,700-year-old clay tablet from Babylonia, it appears that applied mathematics was used to survey land and measure its boundaries with extreme precision. Daniel F Mansfield of the School of Mathematics and Statistics at the University of New South Wales revealed the discovery after studying Si.427, which dates from the Old Babylonian period between 1900 and 1600 BCE. An antiquity object of the highest level of sophistication and interest, the table is part of the Plimpton 322.
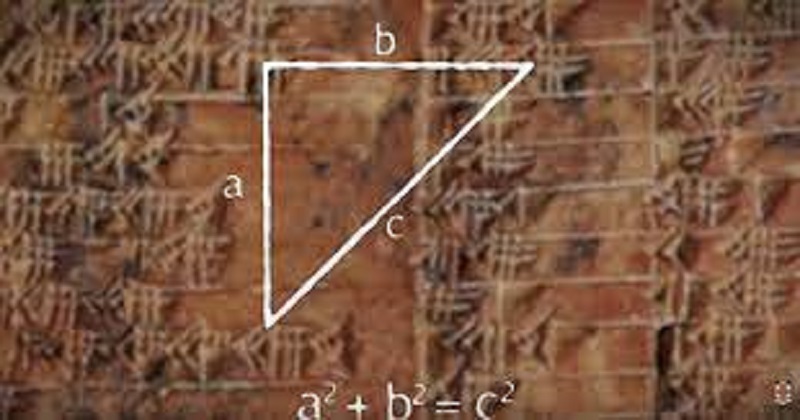
The tablet was discovered in 1894 by French archaeologist Vincent Scheil at Sippar, the site of an ancient Near Eastern Sumerian and later Babylonian city in present-day Iraq. The Si.427 design is considered to be one of the greatest examples of applied geometry from the ancient world, according to Daniel F Mansfield. In a paper published in the journal Foundations of Science, a mathematician states that Mesopotamian mathematics was fundamentally about lists. ‘Numerical calculations in administration, engineering, and surveying were performed by scribes, whose knowledge of mathematics was based on lists,’ he explained.
According to the mathematician, Si.427 was used to survey land intended for selling, and the wedge-shaped indentations on the tablet display a field that has marshy areas. In addition, Mansfield provides an analysis of a table of rectangles with information about which sides are regular and which sides aren’t, which indicates a contemporary interest in rectangles with regular sides in Mesopotamian mathematics.

To measure fields, surveyors used these tables, which were divided into roughly rectangular, trapezoidal, and triangle shapes. Rectangles with unequal sizes indicate that these surveyors used Pythagorean triples to draw perpendicular lines. According to the tablets, the measurements were done with an unusually high amount of precision compared to earlier field plans found from that period. Three Pythagorean triples appear in Si.427: 3, 4, 5; 8, 15, 17; and 5, 12, 13. There are three whole numbers here representing right-angle triangles because the squares of the first two equal the square of the third: 32 + 42 = 52.
Read more: Ex-resident accused of operating sleeper cells for IS
As Pythagoras demonstrated, the square of the hypotenuse equals the sum of the squares of the other two sides of a right triangle (one of which is 90 degrees): a2+b2=c2. It wasn’t until the Pythagorean principles were defined in the 6th century BC that mathematics truly became a science, over 1,000 years after Si.427 was discovered. Mansfield determined Plimpton 322 was a rectangle study and that its author systematically generated 38 rectangles. In conclusion, he said, ‘It could have been motivated by a practical need, or by a purely theoretical interest in geometry’.

Post Your Comments